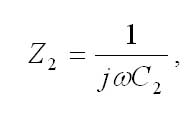Instrumentation for level measurements

An accurate level measurement is crucial for the evaluation of the quantity of materials, phase boundaries, etc. in storage and processing vessels (tanks, wells, reservoirs, columns, etc.). This apparatus may operate under high pressure, under vacuum or at atmospheric pressure at various temperatures. Substances may be as follows: liquids, vapours, gases, solids and their combination. These substances may be corrosive and aggressive. All the above mentioned creates difficulties for level measurement in industrial environment.  In the upcoming posts several widely used instruments will be explained :


 1) Float actuated devices
2) Displacer (buoyancy) devices
3) Liquid head pressure devices
4) Capacitance devices
5) Conductance devices
6) Ultrasonic devices
7) Nucleonic devices

Article Source:: Dr. Alexander Badalyan, University of South Australia

Read more...

Instrumentation for Temperature Measurement

Temperature is a physical quantity that is a measure of hotness and coldness on a numerical scale. It is a measure of the thermal energy per particle of matter or radiation; it is measured by a thermometer, which may be calibrated in any of various temperature scales, Celsius, Fahrenheit, Kelvin, etc.

In kinetic theory and in statistical mechanics, temperature is the effect of the thermal energy arising from the motion of microscopic particles such as atoms, molecules and photons.

In the post 'Temperature : Basics' explains all the basic details related to Temperature. The links below will explain the instrumentation for temperature measurements:

• Temperature scales, temperature units
• Liquid-in-glass thermometers
• Filled thermal systems
• Thermocouples
• Resistance temperature detectors
• Thermistors
• Optical and radiation pyrometers

Read more...

Pressure : Introduction

Pressure is defined as force per unit area. It is usually more convenient to use pressure rather than force to describe the influences upon fluid behavior. The standard unit for pressure is the Pascal, which is a Newton per square meter.

For an object sitting on a surface, the force pressing on the surface is the weight of the object, but in different orientations it might have a different area in contact with the surface and therefore exert a different pressure.

There are many physical situations where pressure is the most important variable. If you are peeling an apple, then pressure is the key variable: if the knife is sharp, then the area of contact is small and you can peel with less force exerted on the blade. If you must get an injection, then pressure is the most important variable in getting the needle through your skin: it is better to have a sharp needle than a dull one since the smaller area of contact implies that less force is required to push the needle through the skin.

When you deal with the pressure of a liquid at rest, the medium is treated as a continuous distribution of matter. But when you deal with a gas pressure, it must be approached as an average pressure from molecular collisions with the walls.

Pressure in a fluid can be seen to be a measure of energy per unit volume by means of the definition of work. This energy is related to other forms of fluid energy by the Bernoulli equation.

Fig 01 : Relationship between absolute, gauge and vacuum pressures.

The section below shows the links that explains the process variable pressure in detail:

• Units of pressure, types of pressure

• “U”-tube liquid filled manometers

• Bourdon and diaphragm gages

• Dead-weight pressure gages

• Piezoelectric pressure transducers

• Capacitance pressure transducers

Read more...

Capacitance pressure transducers

Fig. 14 presents a transducer for sensing and transmitting differential pressure. Pressures to be measured act on isolating diaphragms 1 and 2 and are transmitted through a silicone oil 3, which fills the system, to a sensing diaphragm 4. This sensing diaphragm is balanced by two forces developed by measured pressures and presents the sensitive element. Capacitor plates 5 and 6 detect the position of the sensing diaphragm, which moves to the left or to the right, and, thus, the differential pressure applied to the sensitive element. The change in electric capacitance is electronically amplified and converted to the standard electrical analog or digital output signal, which is directly proportional to the difference of pressures. In order the capacitance transducer be able to measure comparatively low pressures, the device should produce about 25% change in capacitance for a full-scale pressure change. These transducers have low mass and high resolution. However, they are slightly dependent on temperature variation. Newly developed all-silicon capacitive pressure sensors have better thermal stability.

Figure 14. Variable capacitance differential pressure transducer.

Variable separation capacitance sensors have non-linear relationship between electrical capacitance and the movement of the separating membrane according to the formula:

 (84)

where,

C  - the electrical capacitance of the pressure sensor, F (Farad);

ε₀ = 8.85, pF/m - the permittivity of vacuum, 1pF=10^-12 F;

ε  - the relative permittivity of the insulating material between plates of the capacitor, this is the dimensionless parameter;

A - the cross-sectional area of the capacitor plate, m² ;

d  - the distance between the capacitor plates, m;

a  - variation of the distance between the capacitor plates, m.

A three-plate differential version of the capacitive pressure sensor doesn’t have such disadvantage (see Figure 15).

Two fixed plates form two capacitances with the moving separating plate/membrane as follows:

 (85)                  and               

 (86)

Figure 15. Three-plate differential pressure/displacement sensor

Figure 16 shows an a.c. deflection bridge for the detection of variations of capacitances.

Figure 16. a.c. deflection bridge.

In this bridge:

 (87)                     

,(88)        

 ,(89)

where,

Z₁and Z₂ - reactive impedances, Ohm;

Z₃ and Z₄ - resistive impedances, Ohm.

When I_cd = 0, then V_cd is called an open-circuit voltage of the bridge. According to the Kirchoff’s laws we have:

,                       (90)           

                               .                       (91)

Let potential at V_b = 0, then:

                         

        

  

(92)

So, the relationship between V_cd and a is linear.

Article Source:: Dr. Alexander Badalyan, University of South Australia

Read more...

Piezoelectric pressure transducers

The principle of these pressure transducers is based on the well-known phenomenon, that when an asymmetrical crystal is elastically deformed along its specific axes, an electrical charge is developed on its sides. The value of this charge is proportional to the force applied to the crystal, and, therefore, to the pressure under measurement.

Fig. 12 shows piezoelectric crystal circuit. An electrical charge developed on the sides of the crystal is converted into a voltage-type signal using a capacitor. This voltage is proportional to the electrical charge developed, and to the pressure to be measured. Piezoelectric sensors cannot measure static pressures for more than a few seconds, but they have a very quick response when measure dynamic pressures.

Figure 12. Piezoelectric pressure sensor with electrical circuit.

Synthetically developed quartz crystals (barium titanate, lead zirconate) have similar properties as natural single crystal (quartz). But natural quartz still is the perfect material for manufacturing piezoelectric sensitive elements, because it has perfect elasticity and stability, it is insensitive to temperature variations and it has high insulation resistance.

These pressure transducers are used for measurements of hydraulic and pneumatic pulsations, flow instabilities, fuel injection, etc.

Let’s develop a differential equation for this sensor.

  (60)                         

.   (61)

The dynamic behaviour of a piezoelectric element is analysed from mechanical and electrical points of view.

,                

       (62),                 or using             (63)

we can get the following second order differential equation:

           

                                    (64)

or                        

                                             (65)

Finally, we can re-write (65) with variables in deviation form as follows:

        

                            (66)

Equation (66) is a second order linear differential equation for a piezoelectric sensor with variables in deviation form.

After applying the Laplace transform to (66) we get a transfer function for the piezoelectric sensor:

                                                    

                                (67)

A_x                    - cross-sectional area of the piezoelectric sensor in the direction

perpendicular to axis ‘X’, m²;

b                      - charge sensitivity of the crystal to its deformation in the direction

perpendicular to axis ‘X’, C/m;

F_x                     - effective force due to pressure in the direction of axis ‘X’, N;

k                      - stiffness of the crystal is large ≈ 2 x 10⁹, N/m;

K_x                    - steady state gain of the crystal, in other words it is the charge sensitivity

of the crystal to applied pressure , C/Pa;

m                     - mass of the crystal, kg;

q_piez                  - electrical charge developed by the crystal, C;

P                      - pressure acting on the surface perpendicular to axis ‘X’, Pa;

t                       - time, s ;

x                      - deformation of the crystal in the direction of axis ’X’, m;

λ                      - constant (friction coefficient) for the crystal, N*s / m .

Solution to equation (66) will give us the variation of the electrical charge q_piez , developed on the surfaces of a piezoelement as a function of time for a step change in the input variable – measured pressure, P . Now we should measure this electrical charge. For this purpose two metal electrodes are attached to the opposite sides of a piezoelectric crystal. Thus, a capacitor is formed. The value of capacitance of this capacitor can be evaluated as follows:

                                             (68)

where,

C_piez                             - electrical capacitance of the piezoelement, F (Farad);

ε₀ = 8.85, pF / m         - the permittivity of vacuum, 1pF=10^-12 F;

ε                                  - the relative permittivity of the material of the piezoelectric crystal,

this is the dimensionless parameter;

A_x                                - cross-sectional area of the piezoelectric sensor in the direction,

perpendicular to the axis ‘X’, m²;

d                                  - the thickness of the piezoelectric crystal in the direction,

perpendicular to the axis “X”, m.

The relative permittivity, also called dielectric constant, for various piezoelectric materials is given below :

· for quartz (natural piezoelectric material)   ε = 4.5

· for tourmaline (natural piezoelectric material)  ε = 6.6;

· for lead-zirconate-titanate (man-made piezoelectric ceramic material)  ε = 1500;

· for lead metaniobate (man-made piezoelectric ceramic material) ε = 250.

It is also noted in the above mentioned reference, that natural piezoelectric materials have very low charge to force sensitivity, and therefore man-made piezoelectric ceramic materials are used as sensing elements:

· charge sensitivity to force for quartz                                               2.3, pC/N;

· charge sensitivity to force for tourmaline                                        1.9, pC/N or 2.4, pC/N;

· charge sensitivity to force for lead-zirconate-titanate                      265, pC/N;

· charge sensitivity to force for lead metaniobate                              80, pC/N.

We need to develop an electrical circuit which will allow us to convert variations of the capacitance of the piezoelectric sensor into the variation of an easy measurable electrical signal, voltage, for example. Such equivalent electrical circuit was developed, and is named after Norton (see Figure 13)

Figure 13. Norton equivalent electrical circuit for piezoelectric pressure/force

measurements. 1- piezoelectric element, 2– connecting cable, 3– recorder.

The piezoelectric element can be represented as a current source (or a charge generator) which is connected in parallel with a capacitance C_piez . Then, this element is connected to a voltage recorder via connecting cables, which have the capacitance C_cable. A recorder has a resistive load, R_load. The voltage measured across R_load is equal:

  (69)

where, - the impedance of three resistances connected in parallel,Ohm

According to the definition, the capacitance is equal to the ratio of the charge to the voltage across the capacitor plates, according to:

(70)

Let’s consider capacitance C_piez:

  (71)

After differentiating both sides of (71) we can get:

     

       (72)                  or         

 (73)

or, according to the Ohm’s Law,                              

  (74)

Similar we can get                              

 (75)

Substitution of (74) and (75) into (69) will give:

 (76)

where, s = d/dt            - the Laplace operator.

Expressing variables  V_load and I_piez in deviation form and applying the Laplace transform to (76) we can get:

 (77)

The transfer function for the Norton equivalent electrical circuit for piezoelectric pressure/force measurement system (see Figure 13) is as follows:

  (78)

According to the definition:

,           (79). Expressing these variables in deviation form and applying the Laplace transform to (79) we can get:

.               

                                (80)

The transfer function relating current and charge of the piezoelectric sensor is as follows:

.                             

                  (81)

The transfer function relating the voltage V_load and the measured pressure P can be determined as follows:

.                       (82)

After substitution of (67), (78) and (80) into (82) we can get an expression for an overall transfer function of the piezoelectric pressure/force measurement system:

            

Article Source:: Dr. Alexander Badalyan, University of South Australia

Read more...